CylinderAreaParadox
N.B. Le texte de Bachelard dans la section ci-dessus utilise la lettre n pour désigner le nombre de plans et la lettre m pour le nombre de sommets. Dans le texte ci-dessous n donne le nombre de sommets et m le nombre de plans.
Let  be the surface of a cylinder of height
be the surface of a cylinder of height  and radius
and radius  . (
. ( does not include the flat circular ends of the cylinder.) This
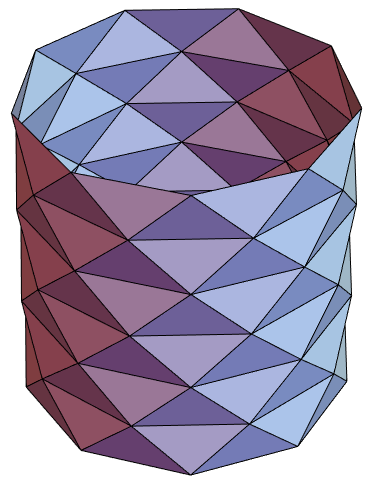
Demonstration constructs a set of triangles that tend uniformly to
does not include the flat circular ends of the cylinder.) This
Demonstration constructs a set of triangles that tend uniformly to  —yet their total area does not tend to the area of
—yet their total area does not tend to the area of  !
!
Divide  into
into  subcylinders (or bands) of height
subcylinders (or bands) of height  . Construct
. Construct  congruent isosceles triangles in each band with vertices at the vertices of a regular
congruent isosceles triangles in each band with vertices at the vertices of a regular  -gon inscribed in the circles at the top and bottom of each band, offset by
-gon inscribed in the circles at the top and bottom of each band, offset by  .
.
For any point  in
in  (except the axis of the cylinder), let
(except the axis of the cylinder), let  be the axial projection of
be the axial projection of  onto
onto  . As
. As  , to say that the triangles approximate
, to say that the triangles approximate  uniformly means that for any point
uniformly means that for any point  on a triangle and any
on a triangle and any  (independent of
(independent of  ), there is a
), there is a  such that for all
such that for all  ,
,  .
.
The sum of the areas of the triangles is
 .
.
Depending on how the limit is taken,  can differ. If first
can differ. If first  with
with  held fixed and then
held fixed and then  , the limit is
, the limit is  , the expected area of the cylinder. If first
, the expected area of the cylinder. If first  with
with  held fixed and then
held fixed and then  , the limit is infinity. If
, the limit is infinity. If  and
and  together so that
together so that  is some positive constant
is some positive constant  , the limit can be chosen to be any number greater than
, the limit can be chosen to be any number greater than  .
.
Therefore  does not have a limit.
does not have a limit.
The surface is known as Schwarz's lantern, Schwarz's polyhedron, or Schwarz's cylinder.
George Beck and Izidor Hafner
"Cylinder Area Paradox"
http://demonstrations.wolfram.com/CylinderAreaParadox/
Wolfram Demonstrations Project